Question

Light of wavelength 530.00 nm is incident normally on a diffraction grating, and the first‑order maximum is observed to be 33.0∘ from the normal. How many slits per millimeter are marked on the grating?

Answer 1

1027.6 lines per mm.

Step-by-step explanation:

wavelength = 530 nm

order, m= 1

Angle = 33 degree

Let the slits per mm is 1/d.

So,

`m \lambda = d sin A\\\\1* 530* 10^(-6) = d sin 33\\\\(1)/(d) = 1027.6 lines per mm`

Answer 2

1028 slits/mm

Step-by-step explanation:

We are given that

Wavelength of light,
`\lambda=530nm=530* 10^(-9) m`

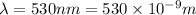
1nm=
`10^(-9) m`

`\theta=33^(\circ)`

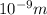
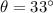
n=1

We have to find the number of slits per mm are marked on the grating.

We know that

`dsin\theta=n\lambda`

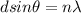
Using the formula

`dsin33^(\circ)=1* 530* 10^(-9)`

`d=(530* 10^(-9))/(sin33^(\circ))`

`d=9.731* 10^(-7) m`

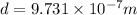
1m=
`10^(3)mm`

`d=9.731* 10^(-7)* 10^3`mm

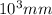
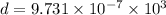`d=0.0009731mm`

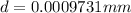
Number of slits=
`(1)/(d)`

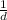
Number of slits=
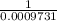`(1)/(0.0009731)`/mm

Number of slits=1028/mm

Hence, 1028 slits/mm are marked on the grating.