Question

If a pendulum's length is 2.00 m and ag = 9.80 m/s, how many complete oscillations does the pendulum make in 5.00 min?​

Answer 1

Number of oscillation = 106 oscillations

Step-by-step explanation:

Given the following data;

• Length = 2 m
• Acceleration due to gravity, g = 9.8 m/s²
• Time = 5 minutes

To find how many complete oscillations the pendulum makes in 5.00 min;

First of all, we would determine the period of oscillation of the pendulum using the following formula;

`T = 2 \pi \sqrt{(l)/(g)}`

Where;

• T is the period.
• l is the length of the pendulum.
• g is acceleration due to gravity.

Substituting into the formula, we have;

`T = 2 * 3.142 \sqrt{(2)/(9.8)}`

`T = 6.284 √(0.2041)`

`T = 6.284 * 0.4518`

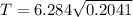
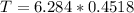
Period, T = 2.84 seconds

Next, we would determine the number of complete oscillation in 5 minutes;

We would have to convert the time in minutes to seconds.

Conversion:

1 minutes = 60 seconds

5 minutes = X seconds

Cross-multiplying, we have;

X = 5 * 60 = 300 seconds

Mathematically, the number of oscillation of a pendulum is given by the formula;

`Number \; of \; oscillation = \frac {Time}{Period}`

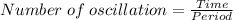
Substituting into the formula, we have;

`Number \; of \; oscillation = \frac {300}{2.84}`

Number of oscillation = 105.63 ≈ 106 oscillations

Number of oscillation = 106 oscillations