Question

Exposure Status Cases Controls Yes 11 108 No 5 436 Total 16 544 Calculate the odds ratio. What do you conclude based upon your results

Answer 1

`Ratio = 8.8`

The exposure may be risk factor.

Step-by-step explanation:

Given

`\begin{array}{ccc}{Exposure\ Status} & {Cases} & {Control} & {Yes} & {11} & {108} & {No} & {5} & {436} & {Total} & {16} & {544} \ \end{array}`

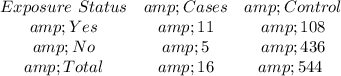
Required

The odd ratio

First, we calculate the odds of exposure using:

`Odds = (With\ Exposure(Yes))/(Without\ Exposure (No))`

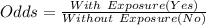
For cases, we have:

`Odds_(Cases) = (11)/(5)`

`Odds_(Cases) = 2.20`

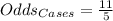
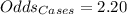
For controls, we have:

`Odds_(Controls) = (108)/(436)`

`Odds_(Controls) = 0.25`

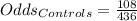
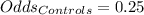
So, the odds' ratio is:

`Ratio = (Odds_(Cases))/(Odds_(Controls))`

`Ratio = (2.20)/(0.25)`

`Ratio = 8.8`

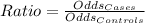
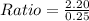
Conclusion about the odds' ratio

The calculated ratio is greater than (and also far from) 1.

This implies that there is a greater exposure than the controls.

So, we can conclude that the exposure may be risk factor.