The question is incomplete. The complete question is :
A mass is attached to the end of a spring and set into oscillation on a horizontal frictionless surface by releasing it from a compressed position. The record of time is started when the oscillating mass first passes through the equilibrium position, and the position of the mass at any time is described by x = (4.7 cm)sin[(7.9 rad/s)πt].
Determine the following:
(a) frequency of the motion
(b) period of the motion
(c) amplitude of the motion
(d) first time after t = 0 that the object reaches the position x = 2.6 cm
Solution :
Given equation : x = (4.7 cm)sin[(7.9 rad/s)πt].
Comparing it with the general equation of simple harmonic motion,
x = A sin (ωt + Φ)
A = 4.7 cm
ω = 7.9 π
a). Therefore, frequency,
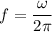
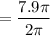
= 3.95 Hz
b). The period,
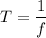
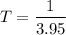
= 0.253 seconds
c). Amplitude is A = 4.7 cm
d). We have,
x = A sin (ωt + Φ)

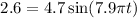
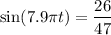

Hence, t = 0.0236 seconds.