Question

If the smallest angle of a triangle is 20° and it is included between sides of 4 and 7, then (to the nearest tenth) the smallest side of the triangle is _____.

Answer 1

`3.5`

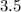
Explanation:

The smallest side of a triangle is formed by the smallest angle in the triangle.

To find the side opposite (formed by) the 20 degree angle, we can use the Law of Cosines. The Law of Cosines states that for any triangle,
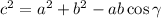`c^2=a^2+b^2-ab\cos \gamma`, where
`a`,
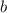`b`, and
`c` are the three sides of the triangle and
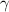`\gamma` is the angle opposite to
`c`.

Let
`c` be the side opposite to the 20 degree angle.

Assign variables:

• 
  `a\implies 4`
• 
  `b\implies 7`
• 
  `\gamma \implies 20^(\circ)`

Substituting these variables, we get:

`c^2=4^2+7^2-2(4)(7)\cos 20^(\circ),\\c^2=16+49-56\cos 20^(\circ),\\c^2=12.377213236,\\c=√(12.377213236)=3.51812638147\approx \boxed{3.5}`

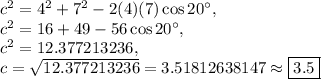
Therefore, the shortest side of this triangle is 3.5.