Question

Consider the quadratic function y = 0.3 (x-4)2 - 2.5
Determine the axis of symmetry, x =

Answer 1

`x=4`

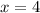
Explanation:

We have the quadratic function:

`\displaystyle y=0.3(x-4)^2-2.5`

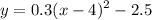
And we want to determine its axis of symmetry.

Notice that this is in vertex form:

`y=a(x-h)^2+k`

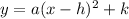
Where (h, k) is the vertex of the parabola.

From our function, we can see that h = 4 and k = -2.5. Hence, our vertex is the point (4, -2.5).

The axis of symmetry is equivalent to the x-coordinate of the vertex.

The x-coordinate of the vertex is 4.

Therefore, the axis of symmetry is x = 4.