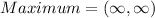Question

What are the maximum and minimum of the function f(x) = 0.9 I -(x - 5) I + 7 ? A. Maximum at (5,7) and minimum at (0,0) B. Minimum at (5,7) and no maximum C. Minimum at (0,0) and no maximum D. Maximum at (5,7) and no minimum

Answer 1

`Minimum = (5,7)`

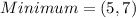
No maximum

Explanation:

Given

`f(x) = 0.9|-(x - 5)| + 7`

Solving (a): The minimum

The minimum is when the absolute parameter gives 0

i.e.

`0.9|-(x - 5)| =0`

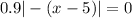
Divide both sides by 0.9

`|-(x - 5)| =0`

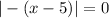
Open bracket

`|-x + 5| =0`

Remove absolute sign

`-x + 5 =0`

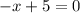
Collect like terms

`x = 5`

Then the y value is:

`f(x) = 0.9|-(x - 5)| + 7`

Recall that:
`0.9|-(x - 5)| =0`

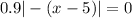
So, we have:

`f(x) = 0 + 7`

`f(x) = 7`

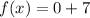
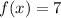
Hence, the minimum is at:
`(5,7)`

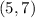
Since the minimum is at
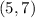`(5,7)`, then the graph will open upwards.

Hence. the function has no maximum; i.e.

`Maximum = (\infty,\infty)`