Question

testing for a disease can be made more efficient by combining samples. If the samples from two people are combined and the mixture tests​ negative, then both samples are negative. On the other​ hand, one positive sample will always test​ positive, no matter how many negative samples it is mixed with. Assuming the probability of a single sample testing positive is 0.15​, find the probability of a positive result for two samples combined into one mixture. Is the probability low enough so that further testing of the individual samples is rarely​ necessary?w./search?q=%E2%80%8B"At+least%E2%80%8B+one"+is+equivalent+to%E2%80%8B+_______.&oq=%E2%80%8B"At+least%E2%80%8B+one"+is+equivalent+to%E2%80%8B+_______.&aqs=chrome..69i57j0i22i30l3.409j0j4&sourceid=chrome&ie=UTF-8 The probability of a positive test result is nothing

Answer 1

(a)
`P(Two\ Positive) = 0.2775`

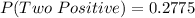
(b) It is not too low

Explanation:

Given

`P(Single\ Positive) = 0.15`

`n = 2`

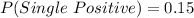
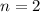
Solving (a):

`P(Two\ Positive)`

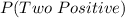
First, calculate the probability of single negative

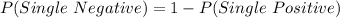`P(Single\ Negative) =1 - P(Single\ Positive)` --- complement rule

`P(Single\ Negative) =1 - 0.15`

`P(Single\ Negative) =0.85`

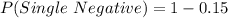
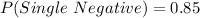
The probability that two combined tests are negative is:

`P(Two\ Negative) = P(Single\ Negative) *P(Single\ Negative)`

`P(Two\ Negative) = 0.85 * 0.85`

`P(Two\ Negative) = 0.7225`

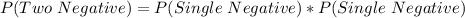
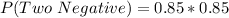
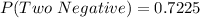
Using the complement rule, we have:

`P(Two\ Positive) = 1 - P(Two\ Negative)`

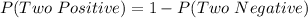
So, we have:

`P(Two\ Positive) = 1 - 0.7225`

`P(Two\ Positive) = 0.2775`

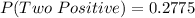
Solving (b): Is (a) low enough?

Generally, when a probability is less than or equal to 0.05; such probabilities are extremely not likely to occur

By comparison:

`0.2775 > 0.05`

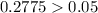
Hence, it is not too low