Question

A bag contains 6 green counters, 4 blue counters and 2 red counters. Two counters are drawn from the bag at random without replacement. Find the probability that no more than one of the counters is blue

Answer 1

24.24%

Explanation:

In other words we need to find the probability of getting one blue counter and another non-blue counter in the two picks. Based on the stats provided, there are a total of 12 counters (6 + 4 + 2), out of which only 4 are blue. This means that the probability for the first counter chosen being blue is 4/12

Since we do not replace the counter, we now have a total of 11 counters. Since the second counter cannot be blue, then we have 8 possible choices. This means that the probability of the second counter not being blue is 8/11. Now we need to multiply these two probabilities together to calculate the probability of choosing only one blue counter and one non-blue counter in two picks.

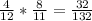`(4)/(12) * (8)/(11) = (32)/(132)` or 0.2424 or 24.24%