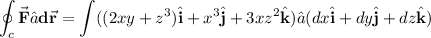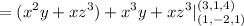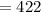Question

F=(2xy +z³)i + x³j + 3xz²k find a scalar potential and work done in moving an object in the field from (1,-2,1) to (3,1,4)​

Answer 1

Explanation:

Given:

`\textbf{F} = (2xy + z^3)\hat{\textbf{i}} + x^3\hat{\textbf{j}} + 3xz^2\hat{\textbf{k}}`

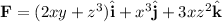
This field will have a scalar potential
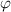`\varphi` if it satisfies the condition
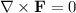`\\abla * \textbf{F}=0`. While the first x- and y- components of
`\\abla * \textbf{F}` are satisfied, the z-component doesn't.

`(\\abla * \textbf{F})_z = \left((\partial F_y)/(\partial x) - (\partial F_x)/(\partial y) \right)`

`\:\:\:\:\:\:\:\:\: = 3x^2 - 2x \\e 0`

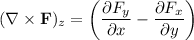
Therefore the field is nonconservative so it has no scalar potential. We can still calculate the work done by defining the position vector
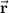`\vec{\textbf{r}}` as

`\vec{\textbf{r}} = x \hat{\textbf{i}} + y \hat{\textbf{j}} + z \hat{\textbf{k}}`

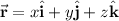
and its differential is

`\textbf{d} \vec{\textbf{r}} = dx \hat{\textbf{i}} + dy \hat{\textbf{j}} + dz \hat{\textbf{k}}`

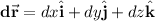
The work done then is given by

`\displaystyle \oint_c \vec{\textbf{F}} • \textbf{d} \vec{\textbf{r}} = \int ((2xy + z^3)\hat{\textbf{i}} + x^3\hat{\textbf{j}} + 3xz^2\hat{\textbf{k}}) • (dx \hat{\textbf{i}} + dy \hat{\textbf{j}} + dz \hat{\textbf{k}})`

`\displaystyle = (x^2y + xz^3) + x^3y + xz^3|_((1, -2, 1))^((3, 1, 4))`

`= 422`