Answer:
Therefore, the standard for will be:
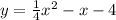
Explanation:
The equation of a parabola written as vertical axes is given by:
 (1)
(1)
The vertex of the parabola (h,k) is (2,-5).
The focus (h,k+p) is (2,-4)
Then we can find p knowing that:
h = 2
k = -5
k + p = -4 then p = -4+5 = 1
Putting all these values in equation (1) we will find the equation of the parabola:
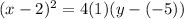
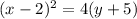 (2)
(2)
Now, we need to find the standard form of the equation of the parabola.
Let's recall that standard form is:
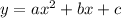
We just need to work out equation (2) to convert it to the standard form.
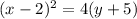
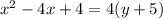
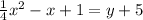
Therefore, the standard for will be:
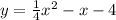
I hope it helps you!