Question

Write an explicit formula for an, the nth term of the sequence 2,8,14

Answer 1

Answer:
`\boldsymbol{a_n = 6n - 4}`

========================================================

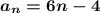
Step-by-step explanation:

`a_1 = 2 = \text{first term}`

`d = 6 = \text{common difference}`

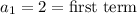
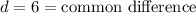
The common difference is 6 because we add 6 to each term to get the next term.

• 2+6 = 8
• 8+6 = 14

Or you can subtract adjacent terms to determine the common difference.

• d = term2-term1 = 8-2 = 6
• d = term3-term2 = 14-8 = 6

Be sure to keep the order of subtraction consistent. Always subtract off the previous term. Note that the sequence is increasing, so the value of d must be positive.

We'll use the values
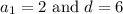`a_1 = 2 \text{ and } d = 6` to determine the nth term formula of this arithmetic sequence.

`a_n = a_1 + d(n-1)\\\\a_n = 2 + 6(n-1)\\\\a_n = 2 + 6n-6\\\\\boldsymbol{a_n = 6n - 4}`

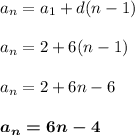
which is the final answer.

Then as partial verification, we can plug in positive integers for n to get corresponding terms.

For instance, plug in n = 3 to find that:

`a_n = 6n - 4\\\\a_3 = 6*3 - 4\\\\a_3 = 18 - 4\\\\a_3 = 14`

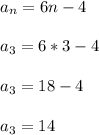
Telling us that the 3rd term is 14, which matches what the instructions mentioned. I'll let you confirm the other terms.