Answer:
Magnetic field strength: approximately
 .
.
Force on the electron: approximately
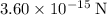 .
.
Step-by-step explanation:
Look up the charge and mass of an electron:
- The magnitude of charge on an electron is the same as the elementary charge:
 .
. - Electron rest mass:
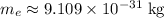 .
.
Since the electron is moving perpendicularly across a magnetic field, magnitude of the magnetic force on this electron would be:
 ,
,
Where:
 is the magnitude of the electric charge on this electron,
is the magnitude of the electric charge on this electron,
 is the speed of the electron, and
is the speed of the electron, and
 is the magnitude of the magnetic field.
is the magnitude of the magnetic field.
At the same time, because the electron is in a centripetal motion, magnitude of the net force on the electron should satisfy:
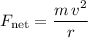 ,
,
Where:
 is the mass of the electron,
is the mass of the electron,
 is the speed of the electron, and
is the speed of the electron, and
 is the radius of the circular orbit.
is the radius of the circular orbit.
Assuming that magnetic force from the field is the only force on this point charge. Net force on the charge would be equal to the magnetic force. In other words:
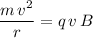 .
.
Rearrange this equation and solve for the magnetic field strength:
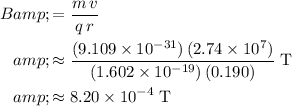 .
.
Substitute
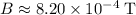 back into the equation
back into the equation
 to find the magnetic force on this electron:
to find the magnetic force on this electron:
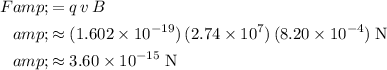 .
.