Question

The diagram shows a 3 cm x 5 cm x 4 cm cuboid

Answer 1

a) The length of segment AC is approximately 5.83 centimeters.

b) The angle ACD is approximately 34.5º.

Explanation:

a) Since
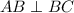`AB \perp BC`, the length of segment
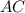`AC` is determined by Pythagorean Theorem, that is:

`AC = \sqrt{(5\,cm)^(2)+(3\,cm)^(2)}`

`AC \approx 5.831\,cm`

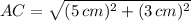
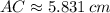
The length of segment AC is approximately 5.831 centimeters.

b) Since
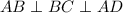`AB \perp BC \perp AD`, the length of segment
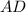`AD` is determined by this Pythagorean identity:

`AD = \sqrt{(3\,cm)^(2)+(5\,cm)^(2)+(4\,cm)^(2)}`

`AD \approx 7.071\,cm`

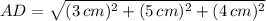
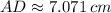
The angle ACD is determined by the following trigonometric expression:

`\cos C = (AC)/(CD)`

`\cos C = (5.831\,cm)/(7.071\,cm)`

`\cos C = 0.825`

`C = \cos^(-1) 0.825`

`C \approx 34.448^(\circ)`

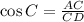
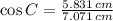
The angle ACD is approximately 34.448º.