Question

Where do the asymptotes occur in

y = 1/3 cot 2x

how can i know?
someone help!

Answer 1

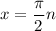`\displaystyle{x=(\pi)/(2)n}` for n is any integers

Explanation:

To know the asymptotes, first, we must know values of x that we turn y-value into an undefined value.

We know that:

`\displaystyle{\cot x = (1)/(\tan x)}`

Now we have to find value of x that turns the identity above into undefined value, and that is
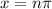`\displaystyle{x=n\pi}` where n is any integers. (This gives 1/0 for all x = nπ)

Therefore, a function
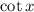`\displaystyle{\cot x}` has asymptote lines at
`\displaystyle{x=n \pi}` for n is integers.

If we consider the given problem:

`\displaystyle{y=(1)/(3)\cot 2x}`

We have to find values of x that turn y-value undefined. We know that
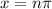`\displaystyle{x=n\pi}` is asymptotes for
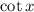`\displaystyle{\cot x}`. Therefore,
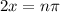`\displaystyle{2x=n\pi}` has to be asymptotes for
`\displaystyle{y=(1)/(3)\cot 2x}`.

Hence, the asymptotes occur at
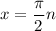`\displaystyle{x=(\pi)/(2)n}` by solving the equation and for n is any integers.