Answer:
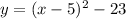
Explanation:
Step 1: Subtract 2 from both sides to get
 .
.
Step 2: Divide B (-10) by 2 to get -5
Step 3: Square your answer to Step 2 to get 25.
Step 4: Use the answer you got to Step 3 as your C value. We get
 .
.
Step 5: Since we added 25 to the right side of the equal sign, we have to add 25 to the left side of the equal sign. We get
 .
.
Step 6: Complete the square, to do this keep the left side of the equal sign the same and change the right side to (x + or - B/2 [depending on if its positive or negative]) squared. In this case it's
 .
.
Step 7: We still have to get our K value because our vertex formula is
 , but in this case our A value is just 1, so it doesn't have to be replaced. So, to get K we subtract 23 from both sides to get our final answer of
, but in this case our A value is just 1, so it doesn't have to be replaced. So, to get K we subtract 23 from both sides to get our final answer of
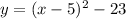 .
.