Question

I need help with this, thx! (Pls show working and steps so I would know how to do other questions!)​

Answer 1

t =
`(1)/(5)`

Explanation:

calculate the gradient (slope) of AB using the slope formula and equate to the slope of the perpendicular line.

given line with slope 1
`(1)/(4)` =
`(5)/(4)`

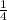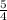
given a line with slope m then the slope of a line perpendicular to it is

`m_(perpendicular)` = -
`(1)/(m)` = -
`(1)/((5)/(4) )` = -
`(4)/(5)`

calculate
`m_(AB)` using slope formula

m =
`(y_(2)-y_(1) )/(x_(2)-x_(1) )`

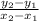
with (x₁, y₁ ) = A (2, - 3 ) and (x₂, y₂ ) = B (- 2, t )

`m_(AB)` =
`(t-(-3))/(-2-2)` =
`(t+3)/(-4)`

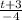
equating corresponding slopes

`(t+3)/(-4)` = -
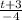`(4)/(5)` ( multiply both sides by - 4 to clear the fraction )

t + 3 = -
`(4)/(5)` × - 4 =
`(16)/(5)` ( subtract 3 from both sides )

t =
`(16)/(5)` - 3 =
`(16)/(5)` -
`(15)/(5)` =
`(1)/(5)`