Question

NO LINKS!! URGENT HELP PLEASE!!!

Find an equation of the circle that has center C(7, -2) and is tangent to the line y = 5

Answer 1

`(x-7)^2+(y+2)^2=49`

Explanation:

A tangent of a circle is a straight line that touches the circle at only one point.

Since the circle is tangent to the horizontal line y = 5, the distance between the center of the circle and the line y = 5 is equal to the radius of the circle.

Given the center of the circle is C(7, -2), the y-coordinate of the center is y = -2. Therefore, the radius of the circle is the difference between y = 5 and y = -2:

`\implies r=5-(-2)=7`

The equation of a circle is:

`\boxed{(x-h)^2+(y-k)^2=r^2}`

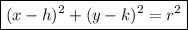
where (h, k) is the center and r is the radius.

Substitute the given center (7, -2) and found radius, r = 7, into the equation of circle:

`(x-7)^2+(y-(-2))^2=7^2`

Simplify:

`(x-7)^2+(y+2)^2=49`

Therefore, the equation of the circle that has center C(7, -2) and is tangent to the line y = 5 is:

`\boxed{(x-7)^2+(y+2)^2=49}`