Question

Which option shows the graph of 2y-10 < 3x?

OA.
OB.
O C.
O D.
←PROBOUS
<-10
10-
10-
10

Answer 1

Answer: B is the correct answer

Step-by-step explanation: I just took the exam :)

Answer 2

Graph B shows the equation 2y-10<3x properly.

Explanation:

See the attached worksheet. Rearrange the equation into standard format oy y = mx+b. where m is the slope and b the y-intercept.

The stadard format is y < (2/3)x + 5

By picking two points on each graph, a slope is calculated. All have a slope of (3/2). The y-intercept is also identified from the graph: again, all have a y-intercept of +5. The only differences in the graphs are therefore the location of the shaded area and the presence of either a dashed or solid line.

Since the equation has the inequality "<," the points satisfying this equation should those that are shaded below the line (Graphs B and C).

And since the inequality is only "<" and not "
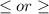`\leq or \geq`," the line should not be solid (it does not include those points actually on the line). A dashed line only appears on Graph B, which also has the correct shading.

Graph B is the correct answer.

The bottom graph is cut off, but all three graphs appear to have the same line: y < (2/3)x + 5. We can eliminate C based on the solid line, without knowing the exact slope.