1 Answer

Ngatirauks · Answer 1 · 2022-12-27T06:13:39+0000

Given:

A figure in which a transversal line intersect two parallel lines.

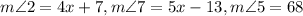$m\angle 2=4x+7, m\angle 7=5x-13, m\angle 5=68$ and
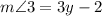$m\angle 3=3y-2$ .

To find:

The value of x and y.

Solution:

We know that, if a transversal line intersect two parallel lines, then

(1) Alternate exterior angles are equal.

(2) Same sided interior angles are supplementary. So their sum is 180 degrees.

In the given figure j and k are parallel lines and l is a transversal line.

From the given figure, it is clear that,

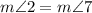$m\angle 2=m\angle 7$ (Alternate exterior angles are equal)

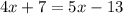
4x+7=5x-13

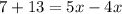
7+13=5x-4x

20=x

Therefore, the value of x is 20.

Now,

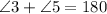$\angle 3+\angle 5=180$ (Same sided interior angles are supplementary)

3y-2+68=180

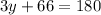
3y+66=180

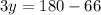
3y=180-66

y=(180-66)/(3)

y=(114)/(3)

y=38

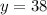
Therefore, the value of y is 38.

Please log in or register to add a comment.