Question

The bar digram represents 24 hours in one day and shows a mark at 30% if sleep of

Answer 1

According to the diagram, the time spent sleeping is 7.2 hours.

How to get the time spent sleeping

Bar graphs are effective for depicting changes over time or making comparisons between different groups. However, they are most effective when the changes being observed are substantial, particularly when measuring changes over time.

Given a bar diagram representing 24 hours in a day with a mark at 30%, it can be represented as:

`\[30\% = (3)/(10)\]`

If this percentage indicates the time spent sleeping, the calculation is:

Time spent sleeping
`\(= 24 \text{ hours} * (3)/(10)\)`

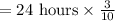
Solving the equation:

`\[= \frac{24 \text{ hours}}{10} * 3\]`

`\[= 2.4 \text{ hours} * 3\]`

`\[= 7.2 \text{ hours}\]`

Therefore, according to the diagram, the time spent sleeping is 7.2 hours.

Question

The bar diagram represents 24 hours in one day and shows a mark at 30%. If you sleep 30% of the day, how many hours do you sleep? Enter your answer in the box.