Answer:
Approximately
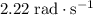 .
.
Step-by-step explanation:
Each revolution increases the angular displacement of the propeller by
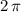 radians. Therefore, after
radians. Therefore, after
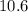 revolutions, the angular displacement of the propeller will be
revolutions, the angular displacement of the propeller will be
 radians.
radians.
Divide angular displacement by the duration to find the angular velocity of this propeller:
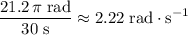 .
.