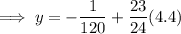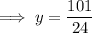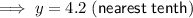Answer:
4.2 (nearest tenth)
Explanation:
Method 1
Draw a scatter plot using the given information.
- The explanatory (independent) variable is the Height and is drawn on the x-axis.
- The response (dependent) variable is Arm Span and is drawn on the y-axis.
Draw a line of best fit (regression line).
To find the Arm Span of a player with a height of 4.4, find y-value of the point of intersection of x = 4.4 and the line of best fit.
Therefore, based on the table, the Arm Span of a player with a height of 4.4 is:
Extrapolation
For this problem, we have used the line of best fit (regression line) to predict a value of that is outside the range of the original data.
When using values of x outside the range to predict corresponding values of y, this is called extrapolation.
These predictions can be unreliable because there is no evidence that the relationship described by the regression line is true for all values of x. Therefore, caution should be applied in these cases.
Method 2
The other way to approach this question would be to enter the data from the table into a statistical calculator and calculate the regression line of y on x:
After entering the data into a statistical calculator:
- a = -8.333333... ×10⁻³
- b = 0.95833333...
Therefore, the equation of the regression line is:

Substitute x = 4.4 into the equation: