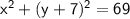Answer:
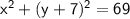
Explanation:
The equation of a circle with center
 and radius
and radius
 is given by the formula:
is given by the formula:

In this case, the center of the circle is
 , and it contains the point
, and it contains the point
 . We can use the distance formula to find the radius (
. We can use the distance formula to find the radius (
 ):
):
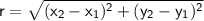
Substitute the coordinates of the center
 and the given point
and the given point
 :
:
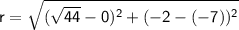
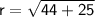
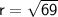
Now, substitute the values of
 ,
,
 , and
, and
 into the circle equation:
into the circle equation:
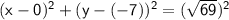
Simplify:
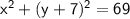
So, the equation of the circle with center
 containing the point
containing the point
 is:
is: