The correct choice is (a) 0 and 1.
To estimate the solution of the system of equations (x + 2y = 5) and (-x + 3y = 6), we can use the given point of intersection, which is approximately (0.6, 2.2) on the coordinate plane.
Let's substitute these values into the two equations:
For
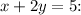
![\[0.6 + 2(2.2) = 0.6 + 4.4 = 5\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/mcajhve9npjwo11fzjcwq5dit4uag4kyia.png)
For
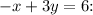
![\[-0.6 + 3(2.2) = -0.6 + 6.6 = 6\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/d0wvp2uq9a6ti8thhhr9213jeknglk60pk.png)
Both equations are satisfied when we use the coordinates of the point of intersection. This verifies that (0.6, 2.2) is indeed a solution to the system.
Now, looking at the x-value of the point of intersection (0.6), we can estimate which integers the x-value is between. Since 0.6 is between 0 and 1, the answer is:
Therefore, the correct choice is (a) 0 and 1.