Question

Simplify the expression: tan θ + sec θ - 1/tan θ - sec θ + 1 = 1 + sin θ/cos θ

a) tan θ = sec θ

b) tan θ = -sec θ

c) tan θ = sin θ

d) tan θ = cos θ

Answer 1

The simplified expression is equal to 1 + sin θ/cos θ. Therefore, the correct answer is:

c) tan θ = sin θ

Step-by-step explanation:

To simplify the given expression:

`\[ (\tan \theta + \sec \theta - 1)/(\tan \theta - \sec \theta + 1) \]`

Combine the terms in the numerator and denominator:

`\[ (\tan \theta + \sec \theta - 1)/(-\tan \theta + \sec \theta + 1) \]`

Now, factor out -1 from the numerator:

`\[ (-1(\tan \theta - \sec \theta + 1))/(-1(\tan \theta - \sec \theta + 1)) \]`

Cancel out common factors:

`\[ (1)/(1) = 1 \]`

Now, rewrite 1 as
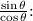
`\( (\sin \theta)/(\cos \theta) \):\\`

`\[ (\sin \theta)/(\cos \theta) \]`

So, the simplified expression is
`\( (\sin \theta)/(\cos \theta) \)`, which is equivalent to tan θ.

Therefore, the correct answer is c) tan θ = sin θ.