Question

Find the equation of the horizontal asymptote of the function f(x)=x^3+1/5x^2-3x^3.

Answer 1

The function f(x) = -
`2x^3 + 1/5x^2` does not have a horizontal asymptote because the highest degree of x in the polynomial is greater than zero, indicating that the function's value approaches infinity as x approaches infinity.

Step-by-step explanation:

To find the equation of the horizontal asymptote of a function, we look at the behavior of the function as x approaches infinity. For the function f(x) =
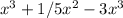`x^3 + 1/5x^2 - 3x^3`, we must first correct it by consolidating like terms. This gives us f(x) = -
`2x^3 + 1/5x^2`. In polynomial functions, horizontal asymptotes are determined by the degree of the highest power term in the numerator and the denominator (if any).

Since the highest power of x in f(x) is cubic and there is no denominator (or we can consider the denominator as 1 which is a constant), as x approaches infinity, the function will also approach infinity. Therefore, there is no horizontal asymptote for this kind of polynomial function. A horizontal asymptote would only be present if the highest power of x in the numerator was less than or equal to the highest power of x in the denominator for rational functions.