Question

The Puck and Pawn Company manufactures hockey sticks and chess sets. Each hockey stick yields an incremental profit of $2 and each chess set, $4. A hockey stick requires 4 hours of processing at machine center A and 2 hours of processing at machine center B. A chess set requires 6 hours at machine center A, 6 hours at machine center B, and 1 hour at machine center C. Machine Center A has a maximum of 120 hours of available capacity per day, machine center B has 72 hours, and machine center C has 10 hours. If the company wishes to maximize profit, how many hockey sticks and chess sets should be produced per day

Answer 1

For number of units of hockey stick = 24

For number of units of chess sets = 4

Maximum possible profit = $64

Step-by-step explanation:

Decision Variables:

Number of units of Hockey sticks and chess sets

Number of Units Hockey Sticks Chess Sets

H C

Objective Function:

Maximize the total profit:

Max P = 2H + 4C

Constraints:

4H + 6C
`\leq` 120 hours ---> A

2H + 6C
`\leq` 72 hours ---->B

C
`\leq` 10 hours -----> C

H, C
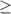`\geq` 0

For this question to solve, we need to draw a feasible region diagram, which I have attached in the attachment. Please refer to it.

So,

Points According to the feasible region are:

D(0,10) ; A(6,10) ; B(24,4) ; C(30,0) ;

Value of objective function at corner points:

At D(0,10) ; P = 2H + 4C = 2x0 + 4 x 10 = $40

At A(6,10); P = 2H + 4C = 2x6 + 4x10 = $52

At B((24,4) : P = 2H + 4C = 2 x 24 + 4x4 = $64

At C(30,0) ; P = 2H +4C = 2x30 + 4x0 = $60

Hence,

P is maximum at corner point B(24,4)

For number of units of hockey stick = 24

For number of units of chess sets = 4

Maximum possible profit = $64