Answer:
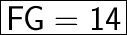
Explanation:
Statement:
Line segment joining the mid-points of two sides of the triangle is parallel and half of the third side.
According to the statement:
CD = 2(FG)
2x + 14 = 2(35 - 3x)
Distribute
2x + 14 = 70 - 6x
Add 6x to both sides
2x + 6x + 14 = 70
8x + 14 = 70
Subtract 14 to both sides
8x = 70 - 14
8x = 56
Divide 8 to both sides
x = 56/8
x = 7
So,
FG = 35 - 3x
FG = 35 - 3(7)
FG = 35 - 21
FG = 14
![\rule[225]{225}{2}](https://img.qammunity.org/qa-images/2023/formulas/biology/high-school/vdifidejf5i8c49g0hg7.png)