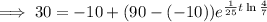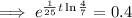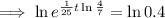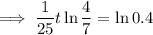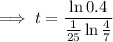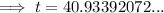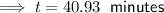Answer:
A) 61.93 minutes
B) 40.93 minutes
Explanation:
Newton's law of cooling

where:
- T(t) is the temperature of the object at time t.
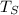 is the temperature of the surrounding environment.
is the temperature of the surrounding environment.
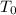 is the initial temperature of the object.
is the initial temperature of the object.- t is time.
- k is a constant.
Given values:
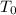 = 90°C
= 90°C
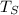 = 20°C
= 20°C- t = 25 minutes
- T(t) = 60°C
Substitute the given values into the formula and solve for k:

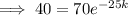
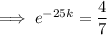
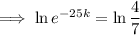
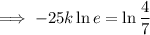
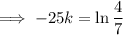
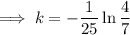
Part A
Given values:
Substitute the given values into the formula and solve for t:
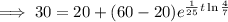
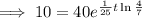
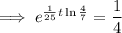
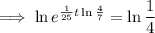
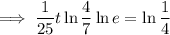
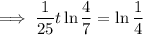
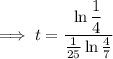
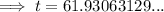
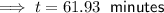
Part B
Given values:
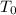 = 90°C
= 90°C
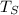 = -10°C
= -10°C
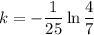
- T(t) = 30°C
Substitute the given values into the formula and solve for t: