Question

Given that the triangles are similar, find the value of x. Find the length of NR.

S
T
40
60
R
2x-2
8
N
M M
X
NR =

Answer 1

Given:

Figure of similar triangles.

To find:

The value of x and the measure of NR.

Solution:

In triangle RST and RMN,

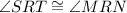`\angle SRT\cong \angle MRN` (Vertically opposite angles)

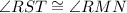`\angle RST\cong \angle RMN` (Alternate interior angles)

`\Delta RST\sim \Delta RMN` (By AA property of similarity)

We know that, corresponding sides of similar triangles are proportional.

`(RS)/(RM)=(RT)/(RN)`

`(40)/(8)=(60)/(2x-2)`

`5=(60)/(2x-2)`

`2x-2=(60)/(5)`

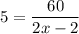
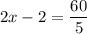
On further simplification, we get

`2x=12+2`

`x=(14)/(2)`

`x=7`

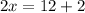
The value of x is 7.

Now,

`NR=2x-2`

`NR=2(7)-2`

`NR=14-2`

`NR=12`

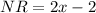
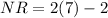
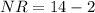
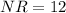
Therefore, the value of x is 7 and the measure of NR is 12 units.