Question

The effectiveness of a blood-pressure drug is being investigated. An experimenter finds that, on average, the reduction in systolic blood pressure is 62.4 for a sample of size 1088 and standard deviation 14.5. Estimate how much the drug will lower a typical patient's systolic blood pressure (using a 98% confidence level). Enter your answer as a tri-linear inequality accurate to one decimal place _______ < μ< _________ ​

Answer 1

In tri-linear inequality format (accurate to one decimal place) is: 61.6 <
`\mu` < 63.2.

To estimate the confidence interval for the mean reduction in systolic blood pressure
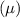`(\(\mu\))`, we can use the formula:

`\[ \text{Confidence Interval} = \text{Sample Mean} \pm \text{Margin of Error} \]`

The margin of error is given by:

`\[ \text{Margin of Error} = Z * \frac{\text{Standard Deviation}}{\sqrt{\text{Sample Size}}} \]`

For a 98% confidence level, the critical z-value is approximately 2.33 (you can find this value from a standard normal distribution table or calculator).

Given values:

- Sample Mean
`(\(\bar{x}\))` = 62.4

- Standard Deviation
`(\(\sigma\))` = 14.5

- Sample Size
`(\(n\))` = 1088

- Critical z-value
`(\(Z\))` for 98% confidence level ≈ 2.33

Now, plug these values into the formulas:

`\[ \text{Margin of Error} = 2.33 * (14.5)/(√(1088)) \]`

Calculate the margin of error.

Then, the confidence interval is:

`\[ 62.4 \pm \text{Margin of Error} \]`

Substitute the calculated margin of error into the formula to get the confidence interval.

The final answer in tri-linear inequality format (accurate to one decimal place) is: 61.6 <
`\mu` < 63.2.