Question

Consider 5.00 L of a gas at 365 mmHg and 20. ∘C . If the container is compressed to 2.30 L and the temperature is increased to 40. ∘C , what is the new pressure, P2, inside the container? Assume no change in the amount of gas inside the cylinder.

Answer 1

P₂ = 1.12 atm

Step-by-step explanation:

To find the new pressure, you need to use the Combined Gas Law:

`(P_1V_1)/(T_1)=(P_2V_2)/(T_2)`

In this equation, "P₁", "V₁", and "T₁" represent the initial pressure, volume, and temperature. "P₂", "V₂", and "T₂" represent the new pressure, volume, and temperature. Before plugging the values into the equation, you need to

(1) convert the pressure from mmHg to atm (760 mmHg = 1 atm)

(2) convert the temperatures from Celsius to Kelvin (°C + 273)

The final answer should have 3 sig figs like the given values.

P₁ = 365 mmHg / 760 = 0.480 atm P₂ = ? atm

V₁ = 5.00 L V₂ = 2.30 L

T₁ = 20°C + 273 = 293 K T₂ = 40°C + 273 = 313 K

`(P_1V_1)/(T_1)=(P_2V_2)/(T_2)` <----- Combined Gas Law

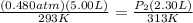`((0.480 atm)(5.00 L))/(293 K)=(P_2(2.30 L))/(313 K)` <----- Insert values

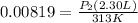`0.00819=(P_2(2.30 L))/(313 K)` <----- Simplify left side

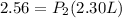`2.56 = P_2(2.30L)` <----- Multiply both sides by 313

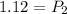`1.12 = P_2` <----- Divide both sides by 2.30