Question

The number of Apple iPods sold in 2009 was 54.13 million. That number decreased approximately linearly to 26.38 million iPods sold in 2013. Let A(t) represent the number of Apple iPods sold (in millions) t years after 2005.

Answer 1

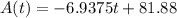`A(t) = -6.9375t + 81.88` is the amount, in millions, for the number of Apple iPods sold in t years after 2005.

Explanation:

Linear function:

A linear function has the following function:

`y = mx + b`

In which m is the slope(the value that y changes by when x changes by 1) and b is the y-intercept(the initial value of the output).

Finding the value in 2005:

This is b.

The number of Apple iPods sold in 2009 was 54.13 million. That number decreased approximately linearly to 26.38 million iPods sold in 2013.

So in 4 years, it decreased from 54.13 - 26.38 = 27.75 million.

So the value in 2005 should also be 27.75 million more than in 2009, that is 54.13 + 27.75 = 81.88. So

`y = mx + 81.88`

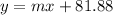
Using A for y and t for x

`A(t) = mt + 81.88`

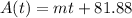
Finding the slope:

When we have two points, the slope is given by the change in the output divided by the change in the input.

In 4 years, the amount decrease by 27.75 million. So

`m = -(27.75)/(4) = -6.9375`

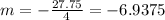
So

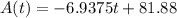`A(t) = -6.9375t + 81.88` is the amount, in millions, for the number of Apple iPods sold in t years after 2005.