Question

Find the perimeter of shape below

​

Answer 1

102.8 cm

Explanation:

The given shape is made up of 4 congruent circles with a diameter of 10 cm, where each circle is tangent to the midpoint of the side of a square with side lengths of 10 cm. Line segments from the endpoints of each diameter are drawn to the vertices (corner points) of the square. (See the attached diagram).

Therefore, the perimeter of the shape consists of the curved part of 4 congruent semicircles (with a diameter of 10 cm) and 8 congruent line segments, each of 5 cm in length.

The length of the curved part of a semicircle is given by πr. Therefore:

`\begin{aligned}\sf Perimeter&=\sf 4* \pi r + 8 * 5\\&=\sf 4 * \pi * 5 + 8 * 5\\&=\sf 20\pi + 40\\&=\sf 102.831853...\; cm\\&=\sf 102.8\; cm\;(nearest\;tenth)\end{aligned}`

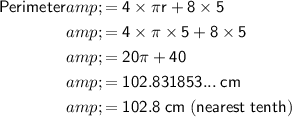
Therefore, the perimeter of the given shape is 102.8 cm (rounded to the nearest tenth).