Final Answer:
Shirley is currently 16 years old.
Step-by-step explanation:
To determine Shirley's age, let's denote her current age as S and Bettina's age as B. The given information states that Bettina is twice as old as Shirley, which can be expressed as B = 2S.
Now, considering the statement that in three years, Bettina will be 13 years less than thrice as old as Shirley, we can create an equation for their ages in three years:
![\[ B + 3 = 3(S + 3) - 13 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/d3xt7kab5fh5s7f98ffhfgpyvtyws6ybzw.png)
Substitute
 into the equation:
into the equation:
![\[ 2S + 3 = 3(S + 3) - 13 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/429d0xayaf1w0jao296ud5n3d1mdj6o5m6.png)
Solve for S:
![\[ 2S + 3 = 3S + 9 - 13 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/r07btotmnakyg60knqd7nnti3h8u40rho7.png)
Combine like terms:
![\[ 2S + 3 = 3S - 4 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/3cyt63mwe0zss2130ohnq2xjpghgcv786c.png)
Subtract 2S from both sides:
![\[ 3 = S - 4 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/tuxqbumon829r0m7e738qo6afqr0jksmc2.png)
Add 4 to both sides:
![\[ S = 7 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/vdqs3zfay2dtc8f8xcfmh1wd4v4mxg4izf.png)
So, Shirley is currently 7 years old. However, remember that the initial question asks for Shirley's age now. To find that, substitute S back into the initial equation
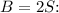
![\[ B = 2(7) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/ybrvyphgkrmn9awo786vyev85gttstl2g4.png)
![\[ B = 14 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/nu004yv4n3j985xd31sqqsvxvz2ksb47ci.png)
Thus, Bettina is currently 14 years old. Therefore, Shirley is 7 years old now.