Question

Find the quotient of (3 x 10⁴) and (6 x 10⁻⁷)

Answer 1

Therefore, the final quotient is
`\((1)/(2) * 10^(11)\)`. In scientific notation, this can be written as
`\(5 * 10^(10)\)`. So, when
`\(3 * 10^4\)` is divided by
`\(6 * 10^(-7)\)`, the result is
`\(5 * 10^(10)\).`

Explanation:

To find the quotient of
`\((3 * 10^4)\)` and
`\((6 * 10^(-7))\)`, you can simply divide the numerical parts and subtract the exponents:

`\[(3 * 10^4)/(6 * 10^(-7)) = (3)/(6) * 10^(4 - (-7)) = (1)/(2) * 10^(11)\]`

So, the quotient is
`\((1)/(2) * 10^(11)\).`

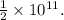
Therefore, the final quotient is
`\((1)/(2) * 10^(11)\)`. In scientific notation, this can be written as
`\(5 * 10^(10)\)`. So, when
`\(3 * 10^4\)` is divided by
`\(6 * 10^(-7)\)`, the result is
`\(5 * 10^(10)\).`