Question

Give each trig ratio as a fraction in simplest form. First find the measure of the missing side using Pythagorean Theorem a2 + b2 = c2

Answer 1

see step-by-step for full solution

Explanation:

First use Pythagoras' Theorem to calculate the length of the missing leg PQ, where a and b are the legs and c is the hypotenuse of a right angled triangle.

a² + b² = c²

⇒ 14² + PQ² = 50²

⇒ 196 + PQ² = 2500

⇒ PQ² = 2500 - 196

⇒ PQ² = 2304

⇒ PQ = √2304 = 48

Therefore, the measure of the missing side PQ is 48.

Now use the 3 basic trigonometry ratios, where O = leg opposite to the angle, A = leg adjacent to the angle, and H = hypotenuse:

`sin(\theta)=(O)/(H) \\\\cos(\theta)=(A)/(H) \\\\tan(\theta)=(O)/(A) \\`

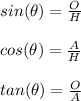
If angle = Q, then O = 14, A = 48, H =50. Therefore,

sin(Q) = O/H = 14/50 = 7/25

cos(Q) = A/H = 48/50 = 24/25

tan(Q) = O/A = 14/48 = 7/24

If angle = R, then O = 48, A = 14, H = 50. Therefore,

sin(R) = O/H = 48/50 = 24/25

cos(R) = A/H = 14/50 = 7/25

tan(R) = O/A = 48/14 = 24/7