Question

Write a slope-intercept equation for a line that passes through (-3,1) and (4, -13).

Answer 1

`\huge{ \bold{y = - 2x - 5}}`

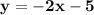
Explanation:

The equation of a line in slope-intercept form is represented by

y = mx + c

where

m is the slope

c is the y-intercept

First of all we have to find the slope from the two points given by using the formula

`m = (y_2 -y_ 1)/(x_2 -x_1 ) \\`

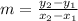
where

(x1, y1) and (x2, y2) are the points

From the question the points are (-3,1) and (4, -13)

We have

`m = ( - 13 - 1)/(4 - - 3) = ( - 13 - 1)/(4 + 3) = - (14)/(7) = - 2 \\`

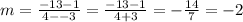
Next we find the y-intercept 'c' by placing one of the points and the slope into the slope-intercept form equation

Using point (-3,1) and m = -2

We have

`1 = ( - 2)( - 3) + c \\ 1 = 6 +c \\ c = 1 - 6 = - 5`

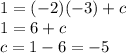
Since we have both m and c we can place it into the main equation to find the equation

Using point and m = -2 c= -5

We have the final answer as

`\bold{y = - 2x - 5}`

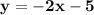
Hope this helps you