Question

Piecharts i havent done this in ages i need for my lil bro

Answer 1

The completed table of values and the pie charts are as follows;

Q1

`\begin{tabular} c \cline{1-3}Colour of Car& Number of Motorists & Angle \\ \cline{1-3}Red & 9 & 81^(\circ) \\\cline{1-3}Yellow &14&106^(\circ) \\\cline{1-3}Blue & 7&63^(\circ) \\\cline{1-3}Other & 10 &90^(\circ)\\\cline{1-3}\end{tabular}`

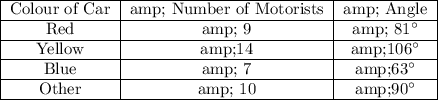
Q2

`\begin{tabular} c \cline{1-3}Product& Amount (\£) &Angle \\ \cline{1-3}Clothes & 49 &148^(\circ) \\\cline{1-3}Games & 21 & 64^(\circ) \\\cline{1-3}Cinema & 14 & 42^(\circ) \\\cline{1-3}Others & 35 &106^(\circ) \\\cline{1-3}\end{tabular}`

The above tables of values are used to draw the required pie charts using MS Excel

The steps to calculate the angles of the sectors formed by the items on the pie chart are as follows;

The angle containing each sector representing the colors of cars on the pie chart can be found as follows;

Red; (9/40) × 360 = 81°

Yellow; (14/40) × 360 = 126°

Blue; (7/40) × 360 = 63°

Other; (10/40) × 360 = 90°

The above values can be used to construct a pie chart using MS Excel

Please find attached the required pie chart created with MS Excel

Q2; The amount spent by Jennifer at the shops indicates that angles occupied on the pie chart are;

Clothes; (49/119) × 360 ≈ 148°

Games; (21/119) × 360 = 64°

Cinema; (14/119) × 360 = 42°

Other; (35/119) × 360 = 106°

The above values can be used to draw a pie chart showing the proportion of the total amount Jennifer spends on each item.

Please find attached the required pie chart, showing the angle of the sectors occupied by Clothes, Games, Cinema, and Others, created with MS Excel