Question

A 4.0 kg particle moves along an x-axis, being propelled by a variable force directed along that axis. Its position is given by: =3.0 m+(5.0 m/s)t+ct² −(6.0 m/s³ )t³

with x in meters and t in seconds. The factor c is a constant. At r=3.0 s the force on the particie has a magnitude of 30 N and is in the negative direction of the axis. What is c ? Units

Answer 1

The constant
`\( c \)` in the particle's position equation
`\( x(t) = 3.0` ,
`\text{m} + (5.0 \, \text{m/s})t` +
`ct^2 - (6.0 \, \text{m/s}^3)t^3 \)` is determined by differentiating the position function to find acceleration
`\( a(t) \)`, then using
`\( F = ma \)` with the given force information at
`\( t = 3.0 \, \text{s} \)`. Solving for
`\( c \)` yields
`\( c = 57.75 \, \text{N/m}^2 \)`.

Let's follow the hint and find the constant
`\( c \)`.

1. Given position function
`\( x(t) = 3.0 \, \text{m} + (5.0 \, \text{m/s})t + ct^2 - (6.0 \, \text{m/s}^3)t^3 \)`

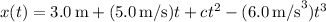
2. Differentiate
`\( x(t) \)` with respect to time
`\( t \)` to get velocity

`\[ v(t) = (dx)/(dt) = 5.0 + 2ct - 18.0t^2 \]`

3. Differentiate
`\( v(t) \)` to get acceleration
`\( a(t) \)`:

`\[ a(t) = (dv)/(dt) = 2c - 36.0t \]`

4. At
`\( t = 3.0 \, \text{s} \)`, the force
`\( F \)` is given by
`\( F = ma \)`, where
`\( m \)` is the mass of the particle and
`\( a(t) \)` is the acceleration.

`\[ F = 4.0 \, \text{kg} \cdot a(3.0) = 30 \, \text{N} \]`

`\[ 30 \, \text{N} = 4.0 \, \text{kg} \cdot (2c - 36.0 \cdot 3.0) \]`

Now, solve for
`\( c \)`:

`\[ 30 \, \text{N} = 4.0 \, \text{kg} \cdot (2c - 108.0) \]`

`\[ 30 \, \text{N} = 8c - 432.0 \, \text{N} \cdot \text{s}^2/\text{m} \]`

`\[ 8c = 462.0 \, \text{N} \cdot \text{s}^2/\text{m} \]`

`\[ c = 57.75 \, \text{N/m}^2 \]`

Therefore, the value of
`\( c \)` is
`\( 57.75 \, \text{N/m}^2 \)`.

Answer 2

The constant c in the particle's position equation is determined by differentiating the position function twice to obtain acceleration, then using F=ma with the given force information at t=3.0 s, resulting in c = 12 N/m².

To determine the constant c, we need to use the information provided. The position of the particle is given by x(t) = 3.0 m + (5.0 m/s)t + ct² - (6.0 m/s³)t³ and the force at t = 3.0 s is 30 N in the negative x-direction. We first find the acceleration by differentiating the position twice with respect to time to obtain the velocity (v(t)) and then the acceleration (a(t)). For the position function, differentiate once to get v(t) = dx/dt = 5.0 m/s + 2ct - 18t² and differentiate again to get a(t) = dv/dt = 2c - 36t. We're interested in force at t = 3.0 s, where the force F is the mass m times the acceleration a. Using F = ma and the given force, 30 N in the negative direction, we set up the equation -30 N = (4.0 kg)(2c - 36(3.0)). Solving for c gives us c = 12 N/m².