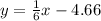Question

Find the equation for the line below

Answer 1

To find the equation of the line we have to find the slope and the intercept so the slope can be found with this equation:

`m=(y_2-y_1)/(x_2-x_1)`

Now we replace two coordinates in the line, so we can see the cordinates (-6,-6) and (4,-4) so:

`\begin{gathered} m=(-4-(-6))/(4-(-6)) \\ m=(2)/(12) \\ m=(1)/(6) \end{gathered}`

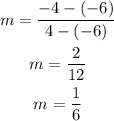
And to find the intercept we replace x=0 and replace one of the coordinates so:

`(1)/(6)=(y-(-4))/(0-4)`

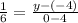
and we solve for y so:

`\begin{gathered} -(4)/(6)=y+4 \\ -(2)/(3)-4=y \\ -4.66=y \end{gathered}`

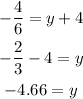
So the equation is:

`y=(1)/(6)x-4.66`