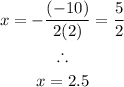Question

Determine whether there is a minimum or maximum value to the quadratic function.f(x) = 2x2 − 10x + 6Find the axis of symmetry.

Answer 1

Maximum or Minimum Value

A quadratic equation is given in the form:

`y=ax^2+bx+c`

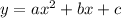
The quadratic graph will open upwards, that is have a minimum value if a > 0 and a maximum value if a < 0.

The quadratic equation in the problem is given to be:

`f(x)=2x^2-10x+6`

Here, a = 2.

Therefore, the graph has a minimum value.

Axis of Symmetry

The axis of symmetry of a parabola is a vertical line that divides the parabola into two congruent halves. It is given by the formula:

`x=-(b)/(2a)`

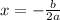
From the equation, b = -10.

Therefore, the axis of symmetry will be:

`\begin{gathered} x=-((-10))/(2(2))=(5)/(2) \\ \therefore \\ x=2.5 \end{gathered}`