Question

A toy car is placed 19.23 cm away from a lens that has a focal length of 5.05 cm. By how much is the car magnified? Where would you need to place an object to make its image appear 15.34 cm away from a lens if the lens has a focal length of 3.16 cm?

Answer 1

In order to find by how much is the car magnified, first let's find the position of the image using the formula below:

`(1)/(f)=(1)/(d_o)+(1)/(d_i)`

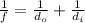
Where f is the focal length, do is the object position and di is the image position.

After converting all measures to meters, we have:

`\begin{gathered} (1)/(0.0505)=(1)/(0.1923)+(1)/(d_i)\\ \\ 19.80198=5.20021+(1)/(d_i)\\ \\ (1)/(d_i)=14.60177\\ \\ d_i=0.0685\text{ m}=6.85\text{ cm} \end{gathered}`

Now, to find the magnification factor, we use the formula below:

`M=(-d_i)/(d_o)=(-0.0685)/(0.1923)=0.356`

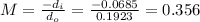
The car is magnified by a factor of 0.356 (that is, the image is smaller than the object)

Now, for the second part of the question, let's use the first formula again, with f = 0.0316 m and di = 0.1534 m:

`\begin{gathered} (1)/(f)=(1)/(d_o)+(1)/(d_i)\\ \\ (1)/(0.0316)=(1)/(d_o)+(1)/(0.1534)\\ \\ (1)/(d_o)+6.5189=31.6456\\ \\ (1)/(d_o)=25.1267\\ \\ d_o=0.0398\text{ m}=3.98\text{ cm} \end{gathered}`

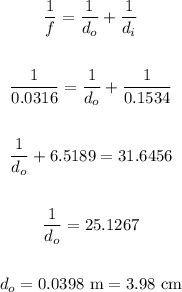
Therefore the object should be put at 3.98 cm.