Question

In AMNO, m M = 24° and mZN = 20°. Which statement about the sides of AMNO must be true? Ο ΜΝ> OM > NO Ο ΟΜΣ MN NO Ο OM NO MN Ο ΝΟΣΟΜΣ ΜΝ Ο ΝΟΣ MN OM Ο ΜΝΣ NOΣ OM

Answer 1

By definition, the Interior angles of a triangle add up 180 degrees. Then:

`m\angle M+m\angle N+m\angle O=180\degree`

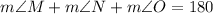
Knowing the measure of the angle M and the measure of the angle N, you can substitute values into the equation and solve for the measure of the angle O. This is:

`\begin{gathered} 24\degree+20\degree+m\angle O=180\degree \\ m\angle O=180\degree-44\degree \\ m\angle O=136\degree \end{gathered}`

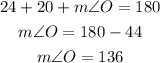
By definition, the largest side of the triangle and the largest angle are opposite to each other and the smallest side and the smallest angle are also opposite. Then, Since you know the angles, you can draw a triangle like the one shown below (it's not drawn to scale):

As you can see, MN is the largest side and the smallest side is OM.

Therefore, you can identify that:

`MN>NO>MO`

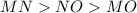
The answer is the last option.