Question

The population of a town in California has been decling by a rate 15% by a rate 15% every year. The population of the was recorded 15,000 in year 2018. Assue that the population of the town will continue to decrease at the same rate. Write exponential equation that represents the declining an provide a brief explanation for your equation

Answer 1

`\text{The answer = P = 15000( 1 - 0.15)}^t`

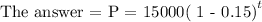
The population is decreasing by 15% every year

The initial population = 15,000

Since it decreases every year

`\begin{gathered} P=a(1+r)^t \\ \text{Where a = initial amount},\text{ r = rate, t = time} \end{gathered}`

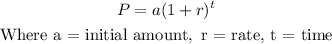
Decreases every year = (100 - 15)

The rate of the population every year = 75%

`P=15,000(1-0.15)^t`

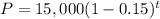
What this simply means is that the population of California keep decreasing by 15%

Assuming the population of California in 2018 is 15000

By 2019 , it would have decreased by 15%

The new population in 2019 will now be = (100 - 15)% x 15000

= 85% x 15000

= 0.85 x 15000

= 12, 750

Therefore, the population in 2019 will now be 12, 750.... It will keep decreasing like that