The inclination is the angle of the line with respect to x-axis:
in our case, angle alpha is equal to 120 degrees:
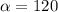
The slope m in the line equation

is related to alpha by the tangent function, that is
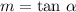
In our case, we have
![\begin{gathered} m=\text{tan 120} \\ \sin ce\text{ tan120=-}\sqrt[]{3,}\text{ it yields} \\ m=-\sqrt[]{3} \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/ic3qq9kzf9jqzesjnvz3.png)
So, our line equation has the form:
![y=-\sqrt[]{3}x+b](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/2bkvmm7b7zrealtho1vq.png)
where b is the y-intercept, which is equal to -6.
Finally, the line equation is
![y=-\sqrt[]{3}x-6](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/vw3ze84j0ny2oyvv0fin.png)