Question

The farmer has 500 feet of fencing to use. What is the maximum possible area the farmer can enclose using all 500 feet of fencing?

Answer 1

Since the length of the fence is 500 feet, then

The perimeter of the rectangle is 500

If the width of the rectangle is x, and the length is y, then

`2x+2y=500`

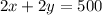
Divide each term by 2

`\begin{gathered} (2x)/(2)+(2y)/(2)=(500)/(2) \\ x+y=250 \end{gathered}`

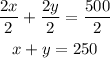
Subtract x from each side to find y in terms of x

`\begin{gathered} x-x+y=250-x \\ y=250-x \end{gathered}`

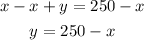
The width of the rectangle is x and the length is 250 - x

Now we can find the area of the rectangle

`\begin{gathered} A=\text{length}* width \\ A=x(250-x) \\ A=x(250)-x(x) \\ A=250x-x^2 \end{gathered}`

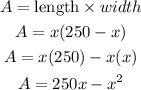
We will find the x-coordinate of the vertex of the quadratic using the rule down

`\begin{gathered} \text{Vertex}\rightarrow(h,k) \\ f(x)=ax^2+bx+c \\ h=(-b)/(2a) \end{gathered}`

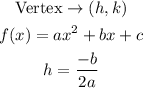
From the equation of the area, we will find a, and b to get h

`\begin{gathered} A(x)=-x^2+250x \\ a=-1 \\ b=250 \\ h=(-250)/(2(-1)) \\ h=(-250)/(-2) \\ h=125 \end{gathered}`

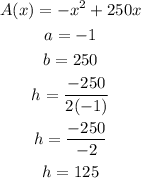
Then the x-coordinate of the vertex is 125 feet which makes the area maximum

Substitute x by 125 in the equation of the area

`\begin{gathered} A=250(125)-(125)^2 \\ A=15625ft^2^{} \end{gathered}`

The maximum area is 15625 square feet