Question

Wyatt earned a score of 45 on Exam A that had a mean of 35 and a standard deviationof 5. He is about to take Exam B that has a mean of 100 and a standard deviation of25. How well must Wyatt score on Exam B in order to do equivalently well as he didon Exam A? Assume that scores on each exam are normally distributed.

Answer 1

Since the scores are normally distributed, we will first obtain the Z score from Exam A, and then use it to find the score required from Exam B to meet that same Z score.

`Z=(X-M)/(\sigma)`

For Exam A, X(score)=45, M(mean)=35, S.D=5

Z will be:

`\begin{gathered} Z=(45-35)/(5) \\ Z=(10)/(5) \\ Z=+2 \end{gathered}`

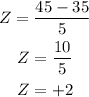
So to obtain, the score (X) for exam B, that will give us the same Z score.

For Exam B, X(score)=unknown, M(mean)=100, S.D= 25

`Z=2=(X-100)/(25)`
`\text{Cross multiply}`
`\begin{gathered} 50=x-100 \\ 50+100=x \\ 150=x \end{gathered}`

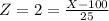
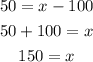
So Wyatt must score 150 in exam B in order to do equivalently well as he

did on exam A.