To fin the length of the sides of rectangle ABCD, we will be using the distance formula.
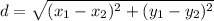
Let's first identify the coordinate of each point.
A (-4, 2)
B (-2, 4)
C (4, -2)
D (2, -4)
The coordinates will be used to substitute for the x's and th y's in the distance formula.
The length of AB is calculated as:
![\begin{gathered} AB=√([-4-(-2)]^2+(2-4)^2) \\ AB=√((-2)^2+(-2)^2) \\ AB=√(4+4) \\ AB=√(8) \\ AB=2√(2) \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/high-school/hdn6xnonkif2r33b8278.png)
Meanwhile, BC's length is:
![\begin{gathered} BC=√((-2-4)^2+[4-(-2)]^2) \\ BC=√((-6)^2+6^2) \\ BC=√(36+36) \\ BC=√(72) \\ BC=6√(2) \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/high-school/92a4mphh6jy5x2xz9hl4.png)
We follow the same steps in calculating hte lengths of CD and AD:
![\begin{gathered} CD=√((4-2)^2+[-2-(-4)]^2) \\ CD=√(2^2+2^2) \\ CD=√(4+4) \\ CD=√(8) \\ CD=2√(2) \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/high-school/70yvdn9eilht7sel1xul.png)
![\begin{gathered} AD=√((-4-2)^2+[2-(-4)]^2) \\ AD=√((-6)^2+6^2) \\ AD=√(36+36) \\ AD=√(72) \\ AD=6√(2) \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/high-school/w0p08oh3ns4z4390zofx.png)
The lengths of the sides of rectangle ABCD are:
AB = 2√2
BC = 6√2
CD = 2√2
AD = 6√2
The area of a recatangle is equal to the product of its length and width.
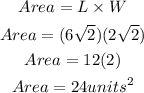
The area of rectangle ABCD is 24 square units.